AMIT 135: Lesson 4 Performance Modeling & Assessment

Contents

Objectives

Upon completing this lesson students should be able to:

Explain the method used to assess the performance of separators.
Illustrate partition analysis for comminution circuit.
Analyze different types of partition curves.
Explain the methods to access separation efficiency from partition curve.

Reading & Lecture

Partition Analysis

One of the most insightful methods for quantifying the performance of separators is a “partition analysis’.
This detailed assessment is commonly performed using a “partition curve’.
Partition curves show the probability of a particular particle having a given characteristics reporting to a given product stream.
Can be achieved for any separation: particle size, density, magnetics, floatability, etc.

Density tracers recovered form floats and sinks immediately show the partition curve. — Density tracers recovered from floats and sinks immediately show the partition curve. [image: (135-4-1)]

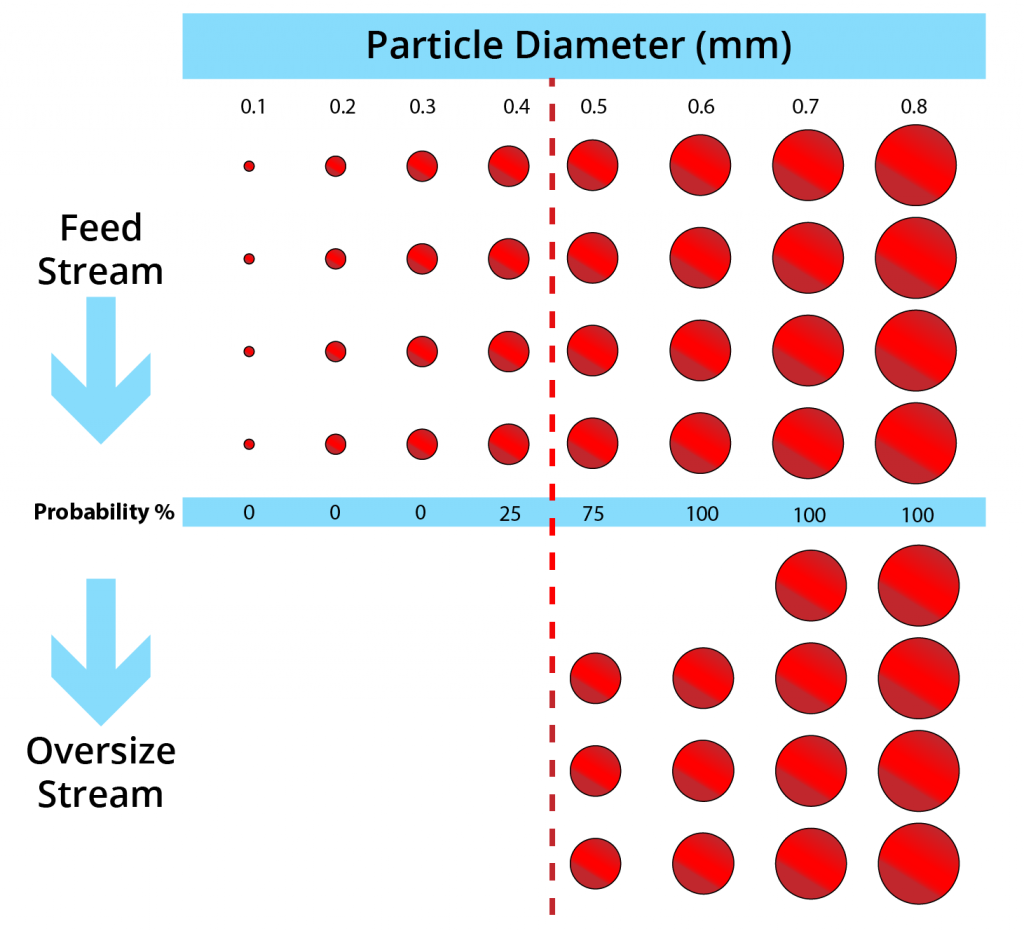
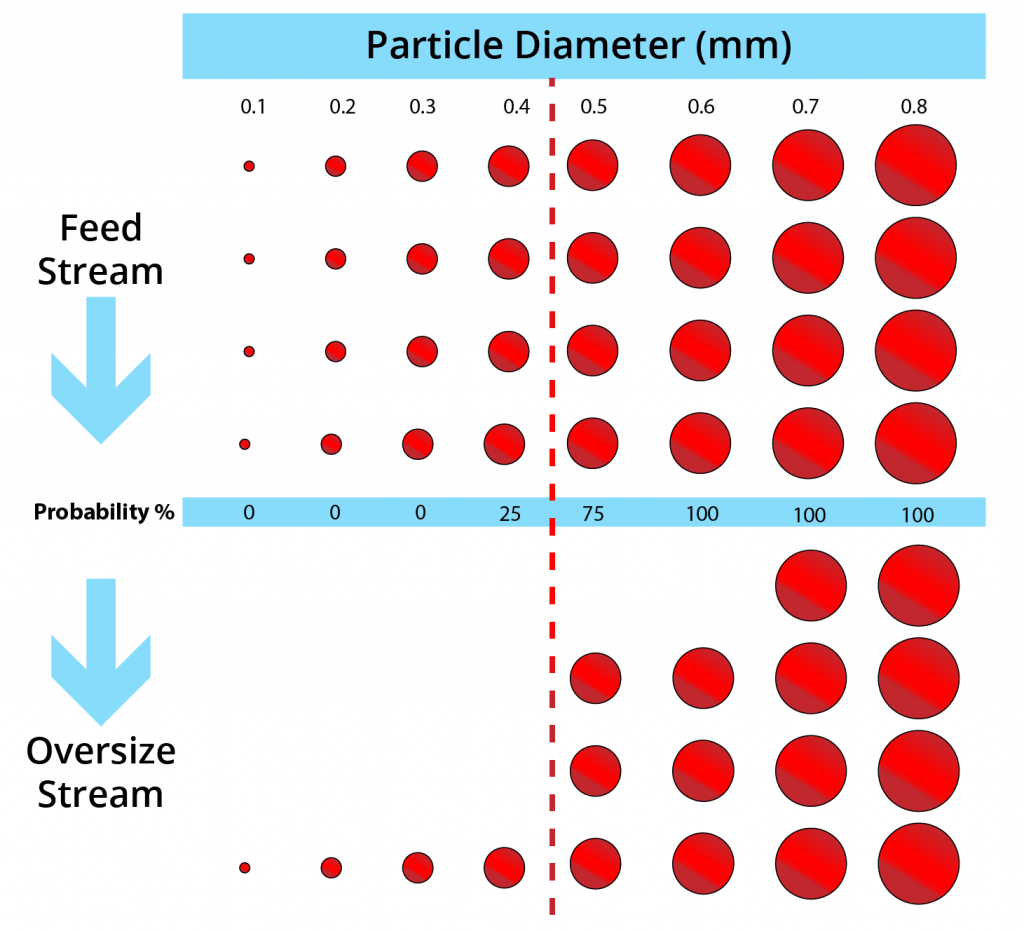
To explain partition curves, let’s take a look at a “perfect’ particle size separation at 0.45 mm.
As shown, 100% of particles > 0.45 mm in each in each size class in the feed report to oversize.

A diagram depicting perfect particle separation
[image: (135-4-2)]

If the separation is less than perfect, then particles can be “misplaced’ to the wrong streams.
This includes:
- Misplacement of coarser particles to undersize
- Misplacement of finer particles to oversize

A diagram illustrating less then perfect particle separation — Less then perfect particle separation
[image: (135-4-3)]

A diagram comparing two separation curves — A Comparison of separation curves
[image: (135-4-4)]

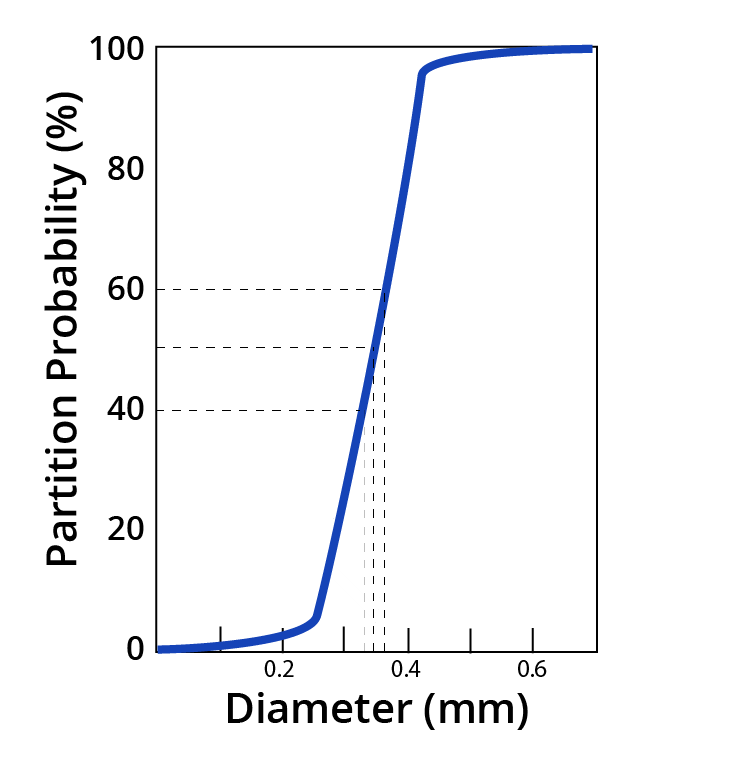
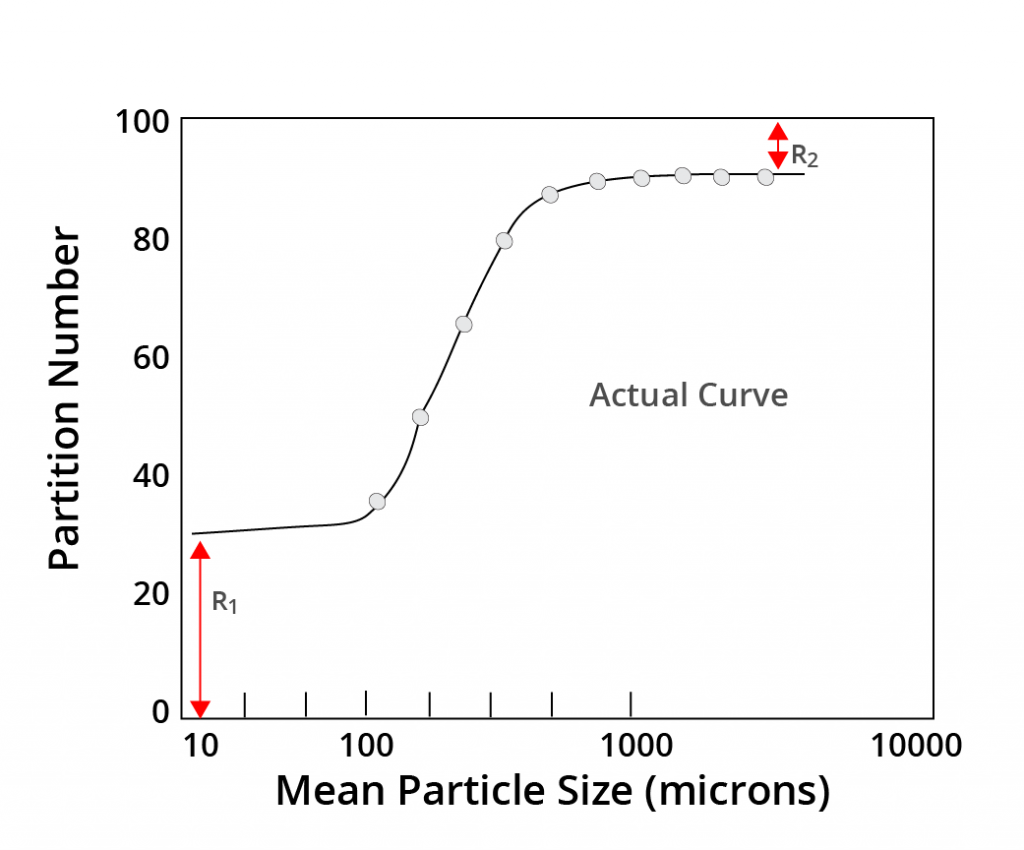
If ideal, curve runs parallel to abscissa at the “cutsize’.
More deviation from axis means more misplaced material.
Shape is characteristic of separator type and operation.

Cut — Point
= D₅₀
= 0.45 mm

Ecart Probability (E_p)
= [D₇₅-D₂₅]/2
=(0.5-0.4)/2=0.05 mmImperfection (I)
=E_P/D₅₀
=0.05/2 = 0.025

Curve shape is an inherent characteristic of the type of separator employed.
Commonly reported values for imperfection range from 0.005 to more than 0.50.
Depends on:
- Type of equipment (e.g., screens, cyclones hydraulic sizers, etc)
- Characteristics of feed material (e.g., particle size, shape, density, etc.)
- Production demands (feed rate, water quality, etc.)

A diagram comparing separation efficiency — A comparison of separation efficiency
[image: (135-4-6)]

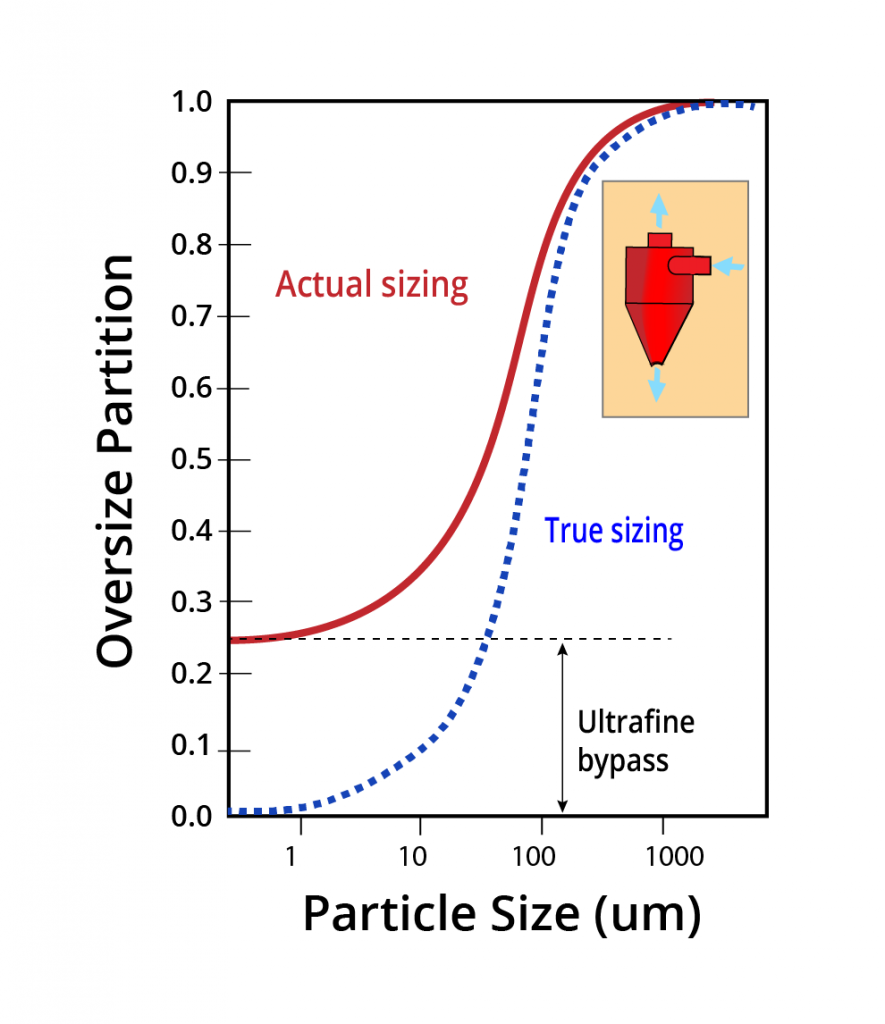
Another important issue is “bypass’.
Bypass can occur to both oversize and undersize.
Oversize bypass is not unusual for screens
Undersize bypass very common for classifiers.

A diagram depicting bypass results
[image: 135-4-7)]

Bypass is the misplacement of fines via entrainment into the oversize product.
Quantified by zero-size offset on the partition curve.
Can sometimes >30 °/o for fine sizing applications.
Bypass typically has a large adverse downstream impact.
Classifiers are often used in multiple stages to reduce bypass (i.e., retreat oversize).

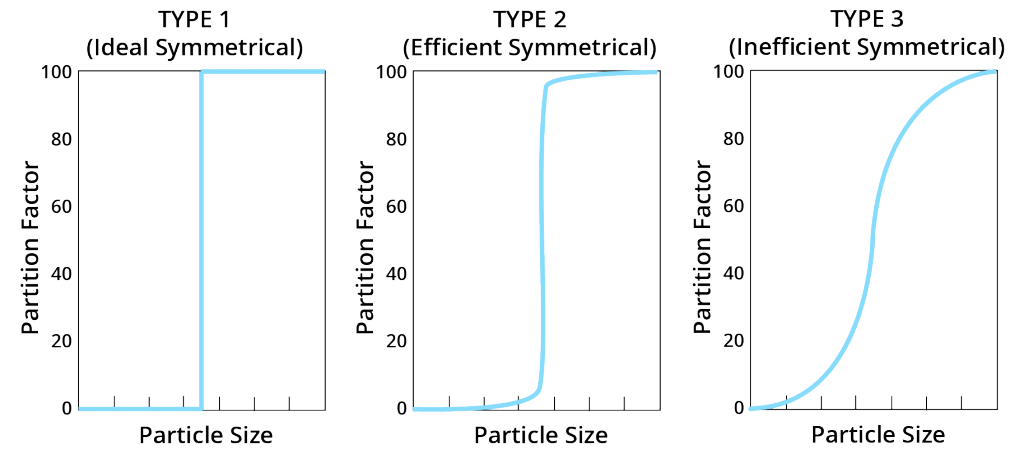
Acceptable Curves:

Type 1- Ideal Symmetrical
- represents perfect processes (e.g., laboratory sieve data)
Type 2 – Efficient Symmetrical
- OK for efficient units (e.g., well designed/operated screen)
Type 3 – Inefficient Symmetrical
- OK for less efficient units (e.g., fine hydraulic classifiers)

Acceptable separation curves
[image: (135-4-9)]

Undesirable Curves:

Type 4- Oversize Nonsymmetrical
- shows loss of coarse to undersize (e.g., holes in screens)

Type 5 – Undersize Nonsymmetrical
- shows loss of fines to oversize (e.g., overloaded screen)

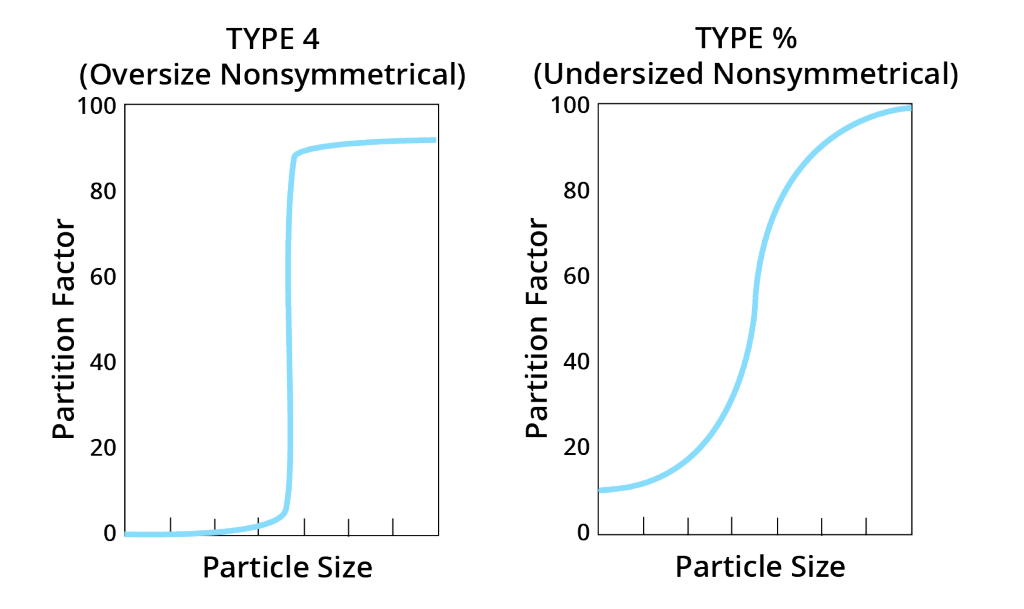
Undesirable separation curves
[image: (135-4-10)]

Reasons:

Inherent unit characteristic, poor circuit design, excessive rates, mechanical failure, poor operating practices, others …

Step 1- Collect Samples

Collect representative samples of the feed, oversize and undersize streams.
Make sure that all streams have been taken into account.

Step 2 – Perform Size Analysis

Perform a laboratory particle size analysis on each sample.
Assess data to make sure that it is reliable (discussed later).

Size Class (Mesh)	Mean Size (mm)	Feed Mass (%)	U/S (%)	O/S (%)
+10	2.404	4.02	0.00	7.85
10x20	1.202	4.68	0.12	11.78
20x28	0.714	13.41	1.88	26.50
28x35	0.505	11.20	3.82	18.10
35x48	0.357	5.03	3.53	7.20
48x65	0.252	10.86	11.52	10.08
65x100	0.178	12.50	16.29	7.19
100x150	0.126	14.27	20.96	5.72
150x200	0.089	8.40	15.30	2.28
200x325	0.058	10.25	17.52	2.16
-325	0.030	5.39	9.06	1.14
		100.0	100.0	100.0

Step 3 – Conduct Calculations

Plot (u-f) versus (u-o).
Data should form a line passing through zero .
Line slope is the fraction of feed tonnage that reports to oversize.
You may disregard unreliable points that do not appear to fall along the line.

Size Class (Mesh)	Mean Size (mm)	Feed Mass (%)	U/S (%)	O/S (%)	X-axis (u-o)	Y-axis (u-f)
+10	2.404	4.02	0.00	7.85	-7.85	-4.02
10x20	1.202	4.68	0.12	11.78	-11.66	-4.56
20x28	0.714	13.41	1.88	26.50	-24.62	-11.53
28x35	0.505	11.20	3.82	18.10	-14.28	-7.38
35x48	0.357	5.03	3.53	7.20	-3.67	-1.50
48x65	0.252	10.86	11.52	10.08	1.44	0.66
65x100	0.178	12.50	16.29	7.19	9.1	3.79
100x150	0.126	14.27	20.96	5.72	15.24	6.6
150x200	0.089	8.40	15.30	2.28	13.02	6.90
200x325	0.058	10.25	17.52	2.16	15.36	7.27
-325	0.030	5.39	9.06	1.14	7.92	3.67
		100.0	100.0	100.0

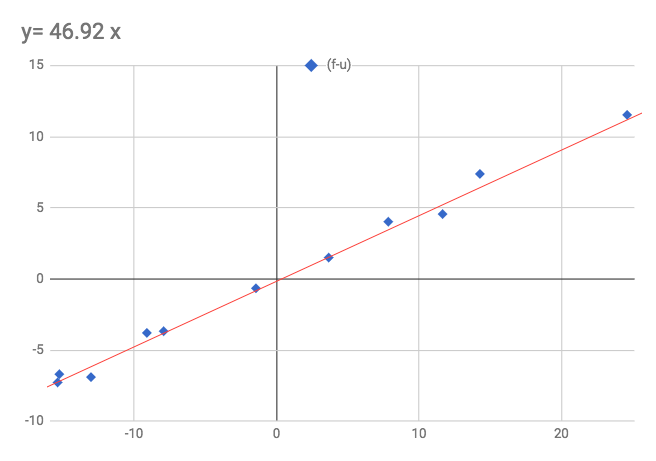
A graph showing line slope partition — Observations? Slope in the first plot shows that 46% of feed reported to oversize.
[image: (135-4-11)]

Step 4 – Construct Partition Curve

Calculate oversize partition for each size class using [(u-f)o]/[(u o)f].

Plot mean size versus partition factor .

Compute performance indicators (cutsize, imperfection, bypass, etc.).

Mean Size (mm)	Feed Mass (%)	U/S (%)	O/S (%)	Y-axis (u-f)	X-axis (u-o)	Percent Feed Weight U/S	Percent Feed Weight O/S	Reconstituted Feed Weight	Partition Number
2.404	4.02	0.00	7.85	4.02	7.85	0.00	3.68	3.68	100.00
1.202	4.68	0.12	11.78	4.56	11.66	0.06	5.53	5.59	98.86
0.714	13.41	1.88	26.50	11.53	24.62	1.00	12.43	13.43	92.57
0.505	11.20	3.82	18.10	7.38	14.28	2.03	8.49	10.52	80.73
0.357	5.03	3.53	7.20	1.50	3.67	1.87	3.38	5.25	64.32
0.252	10.86	11.52	10.08	-0.66	-1.44	6.11	4.73	10.84	43.61
0.178	12.50	16.29	7.19	-3.79	-9.1	8.65	3.37	12.02	28.07
0.126	14.27	20.96	5.72	-6.6	-15.24	11.13	2.68	13.81	19.43
0.089	8.40	15.30	2.28	-6.90	-13.02	8.12	1.07	9.19	11.64
0.058	10.25	17.52	2.16	-7.27	-15.36	9.30	1.01	10.31	9.83
0.030	5.39	9.06	1.14	-3.67	-7.92	4.81	0.53	5.34	10.01
	100.0	100.0	100.0			53.08	46.92	100.00

Yield to Oversize Stream – 46.92%

**Observations?**
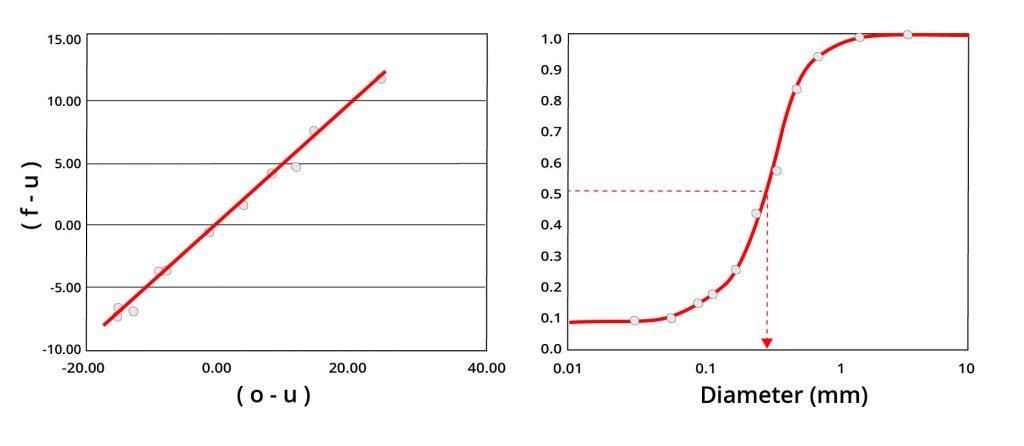
Slope in first plot shows that 46 .9% of feed reported to oversize. Partition curve shows 0.30 mm cutsize, no oversize bypass and 9% undersize bypass. [image: (135-4-12)]

Partition Curve Observations

The partition curve completed for a particle size separation utilized the weight distribution of all process streams including the feed stream.

Depending on the breakage characteristics of the material and the location of the sample points, particle breakage could occur which affects the component balance around the process.

As such, it is sometimes preferred to use component assays (e.g., solid concentration or assays such as iron content) and the two-product equation to determine mass yield.

Using the mass yield, the feed is then reconstituted to determine the partition numbers.

Corrected Partition Number

The equation for determining the corrected Partition Number is:

$Y' = \frac{Y-R_{1}}{1-R_{1}-R_{2}}$

(5-10)
(5-10)

Y’ is the corrected partition number, Y the actual partition number, R₁ , the fractional amount of ultrafines by-passing to the underflow stream and R₂ , the fractional amount of coarsest particles by-passing to the flow stream.

The by-pass of coarse material to the overflow stream is rare but may occur due to a worn vortex finder.
R₂ =0 can be assumed in most cases.

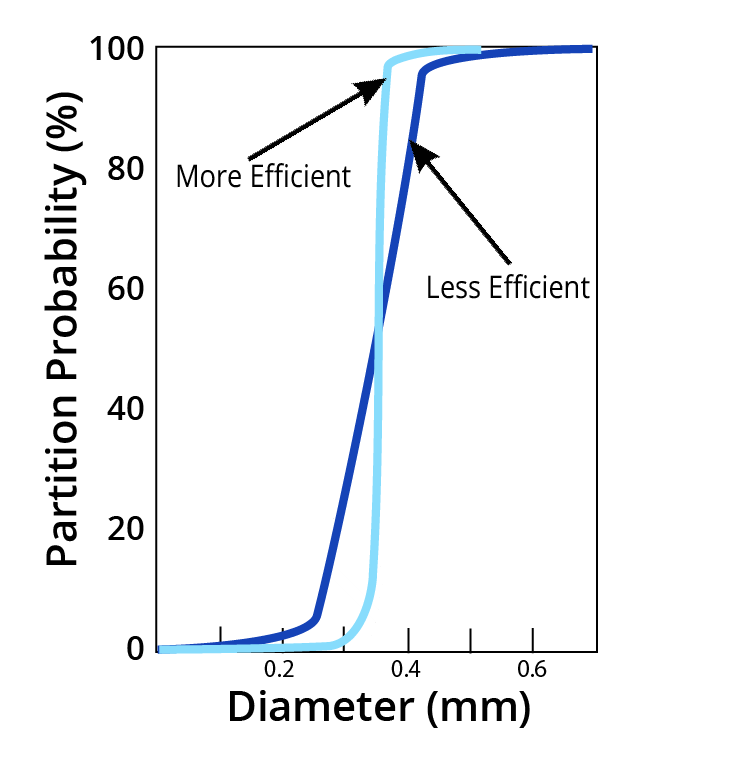
Separation Efficiency

Separation efficiency should always be measured from the corrected partition numbers.

Bypassed particles were not subjected to the separation forces .

For particle size separations, the imperfection value (I) is the preferred measurement:

$I = \frac{d_{75} - d_{25}}{2d_{50}}$

d₇₅, d₅₀ , and d₂₅= the particle size having 75 %, 50% and 25% probabilities, respectively, of reporting to the underflow stream.

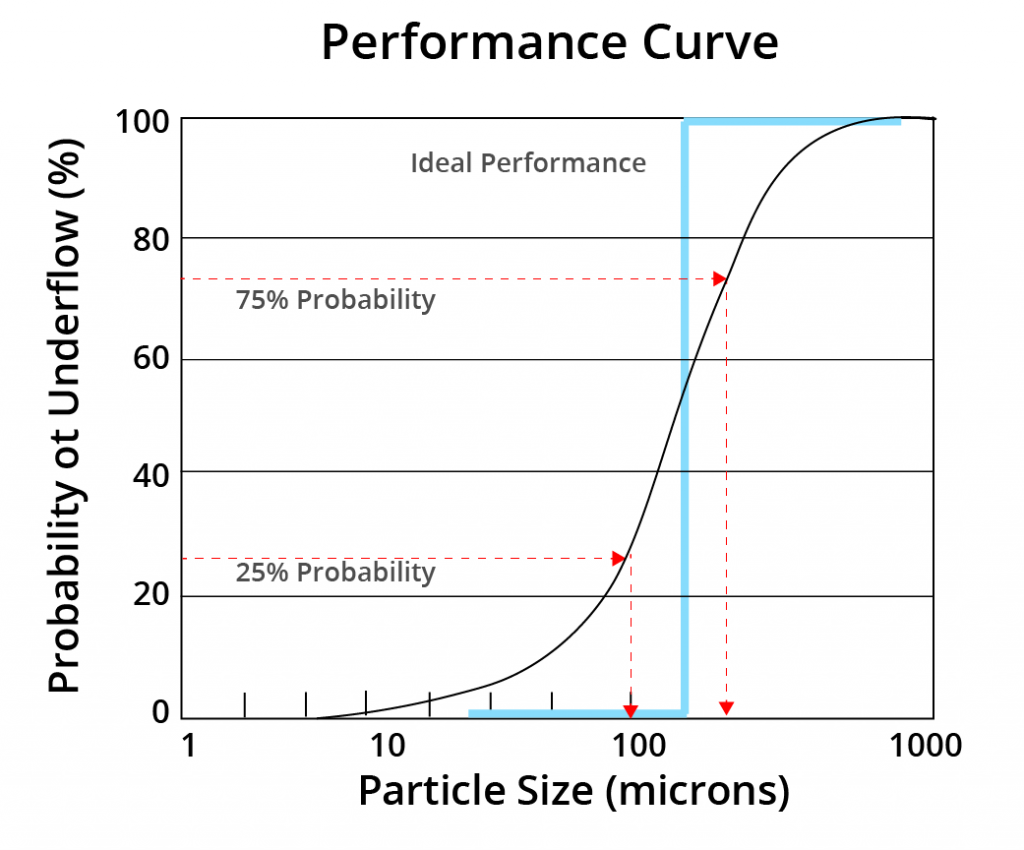
Percent Probability of Underflow
[image: (135-4-14)]

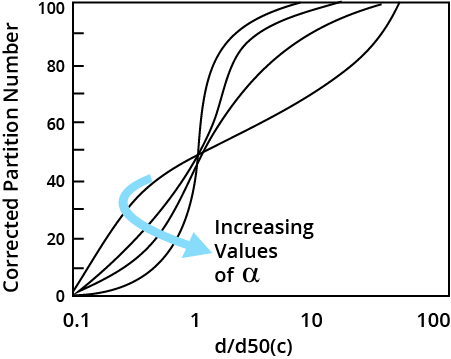
Separation Performance Projection

Many equations are available that model typical performance curves associated with
Lynch and Rao found that the Reduces Efficiency curve can be modeled by the following expression:

$Y' - \frac{exp(\alpha \chi )-1)}{exp(\alpha \chi ) + exp(\alpha )-2))} \; \; \; \; (5-11)$

X= d/d 50(c)

Î± = the curve slope and the value is indicative of the classification efficiency.

The Lynch model can be used to predict the performance of a classifying cyclones.

[image: (135-4-15)] Chart showing increasing values of alpha

Example: Performance Prediction

Given a feed particle size distribution and an alpha value of 2.5, predict the performance of a cyclone. The amount of ultrafine by-pass is assumed to be 20% by weight.

1	2	3	4=Eq.[5-10]	5=Eq.[5-10]	6=2*5	7=6/(∑6)
Mean Particle Size (microns)	Weight (%)	d/d50(c)	Corrected Partition Number	Actual Partition Number	Underflow Weight (%)	Normalized Underflow Weight (%
1200	2.40	12	1.00	1.00	2.40	3.90
850	7.50	8.5	1.00	1.00	7.50	12.18
600	8.90	6	1.00	1.00	8.90	14.45
425	6.40	4.25	1.00	1.00	6.40	10.39
300	6.90	3	1.00	1.00	6.89	11.19
212	4.30	2.12	0.97	0.97	4.19	6.80
150	4.50	1.5	0.82	0.86	3.86	6.28
106	4.00	1.06	0.55	0.64	2.55	4.14
75	3.40	0.75	0.31	0.45	1.52	2.46
53	51.70	0.53	0.17	0.34	17.36	28.20
Total	100.00				61.57	100.00

Mass Yield to Underflow = 61.57%

Summary

“Partition factor” represents the probability that a given particle size in the feed stream will report to the oversize product.
“Partition analysis” makes it possible to determine key performance indicators.
- Cutsize (D50)
- Imperfection (I)
  - Bypass
Plant personnel should monitor and strive to maintain sizing “efficiencies ” since this greatly impacts other plant operations.

Save